Question

What is the correct answer to the question​

Answer 1

4.37%

Explanation:

Given: Value of object in 1997 is $5000

Value of object in 2012 is $4500.

Number of years (2012-1997)= 15 years

∵We know that there is growth in value of object over multiple years.

∴Compound annual growth rate (CAGR)=
`[((end\ value)/(initial\ value) )^{(1)/(n) } ] -1`

Remember, n = number of years

∴ Growth rate=
`[((9500)/(5000) )^{(1)/(15) } ]-1`

⇒Growth rate=
`[(1.9)^{(1)/(15) } -1]`

⇒Growth rate=
`(1.9)^(0.0667) -1= 1.0437-1`

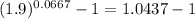
⇒ Growth rate= 0.0437

Now, finding percentage of growth rate

∴
`0.0437* 100= 4.37\%`

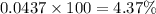
∴ Annual growth percent over the period of time is 4.37%