A car of mass 1,200 kg is travelling along a straight horizontal road at a speed of 20 m/s when it brakes sharply then skids. Friction brings the car to rest. If the coefficient…

Question

asked Sep 24, 2020 114k views

2 Answers

Wenfang Du · Answer 1 · 2020-09-24T19:05:16+0000

Answer:

- 7.84 m/s^2

Step-by-step explanation:

m = 1200 kg

u = 20 m/s

v = 0 m/s

μ = 0.8

the deceleration is goiven by

a = - μ x g - 0.8 x 9.8 = - 7.84 m/s^2

Thus, the deceleration is given by - 7.84 m/s^2.

Psaxton · Answer 2 · 2020-09-29T02:37:53+0000

Answer:

The deceleration of the car is
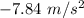
$-7.84\ m/s^2$

Step-by-step explanation:

It is given that,

Mass of the car, m = 1200 kg

Initial speed of the car, u = 20 m/s

Friction brings the car to rest, final speed, v = 0

The coefficient of friction between the tires and road is 0.8

Let a is the deceleration of car due to force of friction. The frictional force is given by :

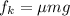
$f_k=\mu mg$

a=-(f_k)/(m)

$a=-(\mu mg)/(m)$

$a=-\mu g$

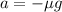
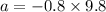
a=-0.8* 9.8

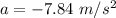
$a=-7.84\ m/s^2$

So, the deceleration of the car is
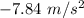$-7.84\ m/s^2$ . Hence, this is the required solution.