Question

The American Water Works Association reports that the per capita water use in a single-family home is 74 gallons per day. Legacy Ranch is a relatively new housing development. The builders installed more efficient water fixtures, such as low-flush toilets, and subsequently conducted a survey of the residences. Twenty-five owners responded, and the sample mean water use per day was 69 gallons with a standard deviation of 8.3 gallons per day. At the 0.05 level of significance, is that enough evidence to conclude that residents of Legacy Ranch use less water on average?

a. What is the decision rule? (Negative amount should be indicated by a minus sign. Round your answer to 3 decimal places.) Reject H0: μ ≥ 74 and fail to reject H1: μ < 74 when the test statistic is .
b. The value of the test statistic is . (Negative amount should be indicated by a minus sign. Round your answer to 2 decimal places.)
c. What is your decision regarding H0? Reject Fail to reject

Answer 1

Reject H0 since test statistic <-2.492

Explanation:

Given that the American Water Works Association reports that the per capita water use in a single-family home is 74 gallons per day.

n = 25

x bar = 69

s= 8.3

std error = s/sqrt n =
`(8.3)/(5) \\=1.66`

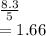
H0:
`\bar x = 74`

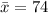
Ha:
`\bar x <74`

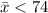
(Left tailed test at 5% significance level)

a) Reject H0 if t
`\leq`-2.492

b) Test statistic = mean difference/std error

=
`(69-74)/(1.66) \\=-3.012\\`

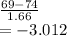
=-3.01

df =24

c) Reject H0