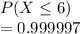Answer:
0.0561,0.0673,0.999997
Explanation:
Given that a sign on the pumps at a gas station encourages customers to have their oil checked, and claims that one out of 8 cars needs to have oil added.
X- no of cars that need oil in a sample of 8 cars is binomial with p = 1/8
since each car is independent of the other and there are only two outcomes.
a) Exactly three out of the next eight cars needs oil.
Probability =
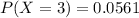
b) At least three out of the next eight cars needs oil.
Probability =
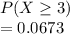
c) At most 6 out of the next 8 cars needs oil.
Probability =