Question

Researchers published a study in which they considered the incidence among the elderly of various mental health conditions such as dementia, bi-polar disorder, obsessive compulsive disorder, delirium, and Alzheimer's disease. In the U.S., 45% of adults over 65 suffer from one or more of the conditions considered in the study. Calculate the probability that fewer than 320 out of the n = 750 adults over 65 in the study suffer from one or more of the conditions under consideration. Give your answer accurate to three decimal places in decimal form. (Example: 0.398)

Probability =

Answer 1

Probability = 0.100.

Explanation:

For each adult, there are only two possible outcomes. Eithey they have one or more of these conditions. Or they do not. This means that the binomial probability distribution is used to solve this problem.

However, we are working with samples that are considerably big. So i am going to aproximate this binomial distribution to the normal.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

Can be approximated to a normal distribution, using the expected value and the standard deviation.

The expected value of the binomial distribution is:

`E(X) = np`

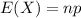
The standard deviation of the binomial distribution is:

`√(V(X)) = √(np(1-p))`

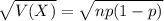
Normal probability distribution

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

When we are approximating a binomial distribution to a normal one, we have that
`\mu = E(X)`,
`\sigma = √(V(X))`.

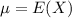In this problem, we have that:

`n = 750, p = 0.45`

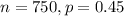
So

`E(X) = 750*0.45 = 337.5`

`√(Var(X)) = √(750*0.45*0.55) = 13.62`

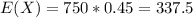
Calculate the probability that fewer than 320 out of the n = 750 adults over 65 in the study suffer from one or more of the conditions under consideration.

This is the pvalue of Z when
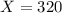`X = 320`. So:

`Z = (X - \mu)/(\sigma)`

`Z = (320 - 337.5)/(13.62)`

`Z = -1.28`

`Z = -1.28` has a pvalue of 0.100.

So

Probability = 0.100.