Question

A uranium nucleus 238U may stay in one piece for billions of years, but sooner or later it decays into an α particle of mass 6.64×10−27 kg and 234Th nucleus of mass 3.88 × 10−25 kg, and the decay process itself is extremely fast (it takes about 10−20 s). Suppose the uranium nucleus was at rest just before the decay. If the α particle is emitted at a speed of 6.31×106 m/s, what would be the recoil speed of the thorium nucleus? Answer in units of m/s

Answer 1

velocity of thorium will be
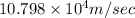`10.798* 10^4m/sec` ( in opposite direction )

Step-by-step explanation:

We have given mass of alpha particle
`m_(\alpha )=6.64* 10^(-27)kg`

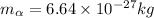
Speed of alpha particle
`v_(\alpha )=6.31* 10^(6)m/sec`

Mass of thorium
`M_(TH)=3.88* 10^(-25)kg`

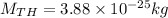
We have to find the velocity of thorium

From conservation of momentum

`M_(\alpha )V_(\alpha )+M_(TH)V_(TH)=0`

`6.64* 10^(-27)*6.31* 10^6+3.88* 10^(-25)* V_(TH)=0`

`V_(TH)=-10.798* 10^4m/sec`

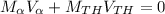
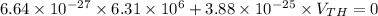
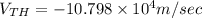
So velocity of thorium will be
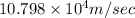`10.798* 10^4m/sec` ( in opposite direction )