Answer:
4820.9 J of work is done on the flight bag.
Step-by-step explanation:
Given:
Force acting on the bag is,
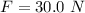
Displacement of the bag caused by the force is,
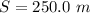
Angle between the direction of force and direction of displacement caused is,

Now, the formula to calculate work done on a body by a force 'F' causing displacement 'S' at an angle
 between the two vectors is given as:
between the two vectors is given as:
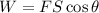
Plug in the given values and solve for work done 'W'. This gives,
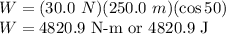
Therefore, the work done on the flight bag is 4820.9 J.