Question

A music industry professional claims that the average amount of money that an average teenager spends per month on music is at least $50. Based upon previous research, the population standard deviation is estimated to be $12.42. The music professional surveys 20 students and finds that the mean spending is $47.77. Is there evidence that the average amount spent by students is less than $50 at a significance level of 0.10?

Answer 1

We conclude average amount of money that an average teenager spends per month on music is at least $50. There is not enough evidence to

Explanation:

We are given the following in the question:

Population mean, μ = $50

Sample mean,
`\bar{x}` = $47.77

Sample size, n = 2-

Alpha, α = 0.10

Population standard deviation, σ = $12.42

First, we design the null and the alternate hypothesis

`H_(0): \mu = 50\text{ dollars}\\H_A: \mu < 50\text{ dollars}`

We use one-tailed(left) z test to perform this hypothesis.

Formula:

`z_(stat) = \displaystyle\frac{\bar{x} - \mu}{(\sigma)/(√(n)) }`

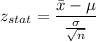
Putting all the values, we have

`z_(stat) = \displaystyle(47.77 - 50)/((12.42)/(√(20)) ) = -0.8025`

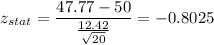
Now,
`z_(critical) \text{ at 0.10 level of significance } = -1.28`

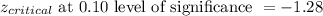
Since,

`z_(stat) > z_(critical)`

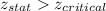
We fail to reject the null hypothesis and accept the null hypothesis. Thus, we conclude average amount of money that an average teenager spends per month on music is at least $50. There is not enough evidence to support the claim.