Question

TV advertising agencies face increasing challenges in reaching audience members because viewing TV programs via digital streaming is gaining in popularity. A poll reported that 55% of 2341 American adults surveyed said they have watched digitally streamed TV programming on some type of device.

(a)

Calculate and interpret a confidence interval at the 99% confidence level for the proportion of all adult Americans who watched streamed programming up to that point in time. (Round your answers to three decimal places.) , Interpret the resulting interval.

We are 99% confident that this interval does not contain the true population proportion.


We are 99% confident that this interval contains the true population proportion.


We are 99% confident that the true population proportion lies below this interval.We are 99% confident that the true population proportion lies above this interval.

Answer 1

a) The 99% confidence interval would be given (0.523;0.577).

We are 99% confident that this interval contains the true population proportion.

b)
`n=(0.55(1-0.55))/(((0.03)/(2.58))^2)=1830.51`

And rounded up we have that n=1831

Explanation:

Data given and notation

n=2341 represent the random sample taken

X represent the people that they have watched digitally streamed TV programming on some type of device

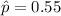`\hat p=0.55` estimated proportion of people that they have watched digitally streamed TV programming on some type of device

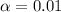`\alpha=0.01` represent the significance level

Confidence =0.99 or 99%

z would represent the statistic for the confidence interval

p= population proportion of people that they have watched digitally streamed TV programming on some type of device

The population proportion present the following distribution:

`p \sim N (p, \sqrt{(p(1-p))/(n)}`

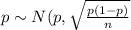
Part a) Confidence interval

The confidence interval would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

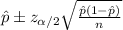
For the 99% confidence interval the value of
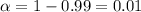`\alpha=1-0.99=0.01` and
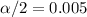`\alpha/2=0.005`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=2.58`

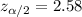
And replacing into the confidence interval formula we got:

`0.55 - 2.58 \sqrt{(0.55(1-0.55))/(2341)}=0.523`

`0.55 + 2.58 \sqrt{(0.55(1-0.55))/(2341)}=0.577`

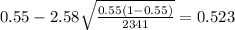
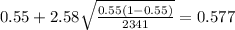
And the 99% confidence interval would be given (0.523;0.577).

We are 99% confident that this interval contains the true population proportion.

Part b) What sample size would be required for the width of a 99% CI to be at most 0.03 irrespective of the value of p??

The margin of error for the proportion interval is given by this formula:

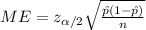`ME=z_(\alpha/2)\sqrt{(\hat p (1-\hat p))/(n)}` (a)

And on this case we have that
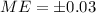`ME =\pm 0.03` and we are interested in order to find the value of n, if we solve n from equation (a) we got:

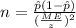`n=(\hat p (1-\hat p))/(((ME)/(z))^2)` (b)

And replacing into equation (b) the values from part a we got:

`n=(0.55(1-0.55))/(((0.03)/(2.58))^2)=1830.51`

And rounded up we have that n=1831