Answer:
Temperature=3.86*10^7K
V1=
 1.4
1.4
Step-by-step explanation:
Deuterium (2H), when heated to sufficiently high tempera- ture, undergoes a nuclear fusion reaction that results in the production of helium. The reaction proceeds rapidly at a temperature, T, at which the average kinetic energy of the deuterium atoms is 8 × 10216 J. (At this temperature, deu- terium molecules dissociate completely into deuterium atoms.)
(a) Calculate T in kelvins (atomic mass of 2H 5 2.015).
(b) For the fusion reaction to occur with ordinary H atoms, the average energy of the atoms must be about 32 × 10216 J. By what factor does the average speed of the 1H atoms differ from that of the 2H atoms of part (a)?
k.E =3/2kT
K.E is the kinetic energy
k=boltzmann constant
T=Temperature in kelvin
juxtaposing the given values we have
T=2(KE)/3k
T=2*8*10^-16/(3*1.38*10^-23)
T=3.86*10^7K
Temperature=3.86*10^7K
b. average speed V=
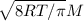
R is the gas constant
T absolute temperature in kelvin
M molar mass
since R.T,PI are constant
we are comparing between hydrogen and helium particles
V=
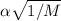
V1/V2=

V1=V2

V1=V2* 1.4
V1=
 1.4
1.4