Answer:

Explanation:
we have that
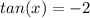
The angle x is in quadrant IV
That means ---> The value of cos(x) is positive and the value of sin(x) is negative
Remember that
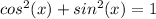 ----> equation A
----> equation A
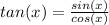
so
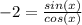
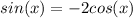 ----> equation B
----> equation B
substitute equation B in equation A
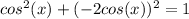
solve for cos(x)
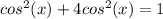
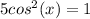
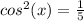
square root both sides
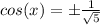
but remember that the value of cos(x) is positive (IV quadrant)
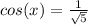
simplify
