Answer:
We conclude that the mean lifetime of a brand of fluorescent bulbs is equal to 1500 hours.
Explanation:
We are given the following in the question:
Population mean, μ = 1500 hours
Sample mean,
 = 1480 hours
= 1480 hours
Sample size, n = 25
Alpha, α = 0.05
Sample standard deviation, s = 80 hours
First, we design the null and the alternate hypothesis

We use one-tailed(left) t test to perform this hypothesis.
Formula:

Putting all the values, we have
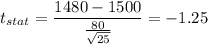
Now,
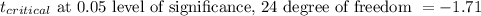
Since,
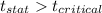
We fail to reject the null hypothesis and accept the null hypothesis.
We conclude that the mean lifetime of a brand of fluorescent bulbs is equal to 1500 hours.