Question

Some sources report that the weights of​ full-term newborn babies in a certain town have a mean of 7 pounds and a standard deviation of 1.2 pounds and are normally distributed.a. What is the probability that one newborn baby will have a weight within 1.2 pounds of the meanlong dashthat ​is, between 5.8 and 8.2 ​pounds, or within one standard deviation of the​ mean?b. What is the probability that the average of nine ​babies' weights will be within 1.2 pounds of the​ mean; will be between 5.8 and 8.2 ​pounds?c. Explain the difference between​ (a) and​ (b).

Answer 1

a)
`P(5.8<X<8.2)=P((5.8-7)/(1.2)<Z<(8.2-7)/(1.2))=P(-1<Z<1)=P(Z<1)-P(Z<-1)=0.841-0.159=0.683`

b)
`P(5.8<\bar X <8.2) = P(Z<3)-P(Z<-3)=0.999-0.0014=0.9973`

c) For part a we are just finding the probability that an individual baby would have a weight between 5.8 and 8.2. So we can't compare the result of part a with the result for part b.

For part b we are finding the probability that the mean of 9 babies (from random sampling) would be between 5.8 and 8.2, so on this case we have a distribution with a different deviation depending on the sample size. And for this reason we have different values

Explanation:

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The Z-score is "a numerical measurement used in statistics of a value's relationship to the mean (average) of a group of values, measured in terms of standard deviations from the mean". The letter
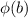`\phi(b)` is used to denote the cumulative area for a b quantile on the normal standard distribution, or in other words:
`\phi(b)=P(z<b)`

Let X the random variable that represent the weights of​ full-term newborn babies of a population, and for this case we know the distribution for X is given by:

`X \sim N(7,1.2)`

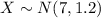
a. What is the probability that one newborn baby will have a weight within 1.2 pounds of the meanlong dashthat ​is, between 5.8 and 8.2 ​pounds, or within one standard deviation of the​ mean?

We are interested on this probability

`P(5.8<X<8.2)`

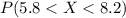
And the best way to solve this problem is using the normal standard distribution and the z score given by:

`Z=(x-\mu)/(\sigma)`

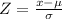
If we apply this formula to our probability we got this:

`P(5.8<X<8.2)=P((5.8-\mu)/(\sigma)<(X-\mu)/(\sigma)<(8.2-\mu)/(\sigma))`

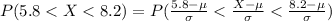
And in order to find these probabilities we can find tables for the normal standard distribution, excel or a calculator.

`P(5.8<X<8.2)=P((5.8-7)/(1.2)<Z<(8.2-7)/(1.2))=P(-1<Z<1)=P(Z<1)-P(Z<-1)=0.841-0.159=0.683`

b. What is the probability that the average of nine ​babies' weights will be within 1.2 pounds of the​ mean; will be between 5.8 and 8.2 ​pounds?

And let
`\bar X` represent the sample mean, the distribution for the sample mean is given by:

`\bar X \sim N(\mu,(\sigma)/(√(n)))`

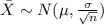
On this case
`\bar X \sim N(7,(1.2)/(√(9)))`

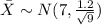
The z score on this case is given by this formula:

`z=(\bar x-\mu)/((\sigma)/(√(n)))`

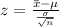
And if we replace the values that we have we got:

`z_1=(5.8-7)/((1.2)/(√(9)))=-3`

`z_2=(8.2-7)/((1.2)/(√(9)))=3`

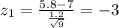
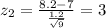
For this case we can use a table or excel to find the probability required:

`P(5.8<\bar X <8.2) = P(Z<3)-P(Z<-3)=0.999-0.0014=0.9973`

c. Explain the difference between​ (a) and​ (b).

For part a we are just finding the probability that an individual baby would have a weight between 5.8 and 8.2. So we can't compare the result of part a with the result for part b.

For part b we are finding the probability that the mean of 9 babies (from random sampling) would be between 5.8 and 8.2, so on this case we have a distribution with a different deviation depending on the sample size. And for this reason we have different values