Question

The times that a cashier spends processing individual customer’s order are independent random variables with mean 2.5 minutes and standard deviation 2 minutes. What is the approximate probability that it will take more than 4 hours to process the orders of 100 people?

Answer 1

0.6915 is the probability that it will take more than 4 hours to process the orders of 100 people.

Explanation:

We are given the following information in the question:

Mean, μ = 2.5 minutes

Standard Deviation, σ = 2 minutes

Since the sample size is large, by central limit theorem, the distribution of sample means is approximately normal.

`P(\sum x_(i) > 4)\\P(\sum x_i > 4* 60\text{ minutes})\\\\P(\displaystyle(1)/(100)\sum x_i > (4* 60)/(100)\text{ minutes})\\\\P(\bar{x} > 2.4)`

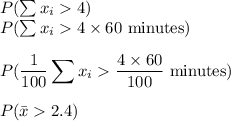
Formula:

`z_(score) = \displaystyle(x-\mu)/((\sigma)/(√(n)))`

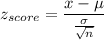
P(it will take more than 4 hours to process the orders of 100 people)

P(x > 2.4)

`P( x > 2.4) = P( z > \displaystyle(2.4-2.5)/((2)/(√(100)))) = P(z > -0.5)`

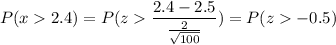
Calculation the value from standard normal z table, we have,
`P(x > 2.4) = 1 - 0.3085 = 0.6915= 69.15\%`

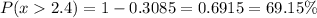
0.6915 is the probability that it will take more than 4 hours to process the orders of 100 people.