Question

The amounts of time that customers stay in a certain restaurant for lunch is normally distributed with a standard deviation of 17 minutes. A random sample of 50 lunch customers was taken at this restaurant. Construct a 99% confidence interval for the true average amount of time customers spend in the restaurant for lunch. Round your answers to two decimal places and use ascending order.

Answer 1

44.89 - 57.27

Answer 2

99% CI: [45.60; 58.00]min

Explanation:

Hello!

Your study variable is:

X: Time a customer stays in a certain restaurant. (min)

X~N(μ; σ²)

The population standard distribution is σ= 17 min

Sample n= 50

Sample mean X[bar]= 51.8 min

Sample standard deviation S= 27.68

You are asked to construct a 99% Confidence Interval. Since the variable has a normal distribution and the population variance is known, the statistic to use is the standard normal Z. The formula to construct the interval is:

X[bar] ±
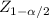`Z_(1-\alpha /2)`*(σ/√n)

`Z_(1-\alpha /2) = Z_(0.995)= 2.58`

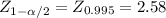
Upper level: 51.8 - 2.58*(17/√50) = 45.5972 ≅ 45.60 min

Lower level: 51.8 + 2.58*(17/√50) = 58.0027 ≅58.00 min

With a confidence level of 99%, you'd expect that the interval [45.60; 58.00]min will contain the true value of the average time customers spend in a certain restaurant.

I hope you have a SUPER day!

PS: Missing Data in the attached files.