Answer: The mass difference between the two is 7.38 grams.
Step-by-step explanation:
To calculate the number of moles, we use the equation given by ideal gas follows:

where,
P = pressure = 125 psi = 8.50 atm (Conversion factor: 1 atm = 14.7 psi)
V = Volume = 855 mL = 0.855 L (Conversion factor: 1 L = 1000 mL)
T = Temperature =
![25^oC=[25+273]K=298K](https://img.qammunity.org/2020/formulas/physics/high-school/h3swi627jfkpg7vx7in8p5pe35bz1gwehq.png)
R = Gas constant =
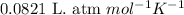
n = number of moles = ?
Putting values in above equation, we get:

To calculate the number of moles, we use the equation:
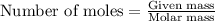 .....(1)
.....(1)
Moles of air = 0.297 moles
Average molar mass of air = 28.8 g/mol
Putting values in equation 1, we get:
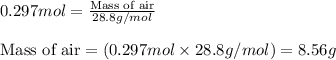
Mass of air,
 = 8.56 g
= 8.56 g
Moles of helium = 0.297 moles
Molar mass of helium = 4 g/mol
Putting values in equation 1, we get:

Mass of helium,
 = 1.18 g
= 1.18 g
Calculating the mass difference between the two:
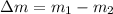

Hence, the mass difference between the two is 7.38 grams.