Question

Find the distance between each pair of points. (-2, 3) and (-7,-7)

Answer 1

`~~~~~~~~~~~~\textit{distance between 2 points} \\\\ (\stackrel{x_1}{-2}~,~\stackrel{y_1}{3})\qquad (\stackrel{x_2}{-7}~,~\stackrel{y_2}{-7})\qquad \qquad d = √(( x_2- x_1)^2 + ( y_2- y_1)^2) \\\\\\ d=√((~~-7 - (-2)~~)^2 + (~~-7 - 3~~)^2) \implies d=√((-7 +2)^2 + (-7 -3)^2) \\\\\\ d=√(( -5 )^2 + ( -10 )^2) \implies d=√( 25 + 100 ) \implies d=√( 125 )\implies d\approx 11.18`

Answer 2

Exact Distance =
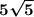`\boldsymbol{5√(5)}` units

Approximate Distance = 11.1803 units

===================================================

Work Shown:

`(x_1,y_1) = (-2,3) \text{ and } (x_2, y_2) = (-7,-7)\\\\d = √((x_1 - x_2)^2 + (y_1 - y_2)^2)\\\\d = √((-2-(-7))^2 + (3-(-7))^2)\\\\d = √((-2+7)^2 + (3+7)^2)\\\\d = √((5)^2 + (10)^2)\\\\d = √(25 + 100)\\\\d = √(125)\\\\d = √(25*5)\\\\d = √(25)*√(5)\\\\d = 5√(5)\\\\d \approx 11.1803\\\\`

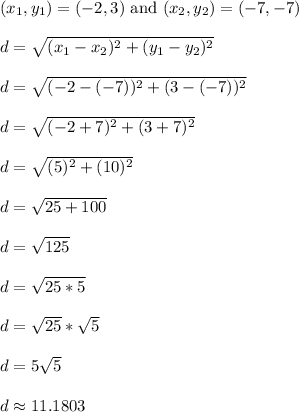
The exact distance is
`5√(5)` units, which approximates to about 11.1803 units

I used the distance formula. Round the approximate value however your teacher instructs.

A slight alternative is to plot the two points to form a right triangle. The hypotenuse goes from (-2,3) to (-7,-7). Then use the pythagorean theorem.

You can use tools like WolframAlpha or GeoGebra to confirm the answer.