Question

A simple pendulum with a length of 2.23 m and a mass of 6.74 kg is given an initial speed of 2.06 m/s at its equi- librium position. Assume it undergoes simple harmonic motion. Determine (a) its period, (b) its total energy, and (c) its maximum angular displacement.

Answer 1

a) T = 2.997 s

b) K = 14.3 J

c) φ = 0.444 rad

Step-by-step explanation:

a) Determine its period

The pendulum simple’s period is:

T = 2π
`\sqrt{(l)/(g) }`

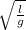
Where l: Pendulum’s length

g = 9.8 m/s2

T = 2π
`\sqrt{(2.23)/(9.8) }`

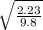
T = 2.997 s

b) Total energy

Initially his total energy is kinetic

K =
`(mv^(2) )/(2)`

K =
`((6.74)(2.06)^(2) )/(2)`

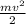
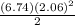
K = 14.3 J

c) Maximum angular displacement

φ =
`cos^(-1)(1-(E)/(mgl) )`

φ =
`cos^(-1)(1-(14.3)/((6.74)(9.8)(2.23)) )`

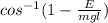
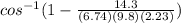
φ = 0.444 rad