Question

A professor is trying to determine if her students guessed on a certain multiple choice question. She expects that if the students guessed, the distribution of answers would be uniform for that question. She compares the observed distribution of answers with the uniform distribution. The professor conducts a chi-square Goodness-of-Fit hypothesis test at the 5% significance level.

a) The null and alternative hypotheses are: H0: The student answers have the uniform distribution. Ha: The student answers do not have the uniform distribution.
b) χ20=13.167.
c) χ20.05=7.815.
d) What conclusions can be made?

Select all that apply:
A. We should reject H0.
B. We should not reject H0.
C. At the 5% significance level, there is sufficient evidence to conclude that the students were not guessing.
D. At the 5% significance level, there is not enough evidence to conclude that the students were not guessing.

Answer 1

A. We should reject H0.

C. At the 5% significance level, there is sufficient evidence to conclude that the students were not guessing.

Explanation:

A chi-square goodness of fit test "determines if a sample data matches a population".

A chi-square test for independence "compares two variables in a contingency table to see if they are related. In a more general sense, it tests to see whether distributions of categorical variables differ from each another".

We need to conduct a chi square test in order to check the following hypothesis:

H0: The student answers have the uniform distribution.

H1: The student answers do not have the uniform distribution.

The level os significance assumed for this case is
`\alpha=0.05`

The statistic to check the hypothesis is given by:

`\chi^2 =\sum_(i=1)^n ((O_i -E_i)^2)/(E_i)`

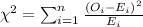
The table given represent the observed values, we just need to calculate the expected values with the following formula
`E_i = (total col * total row)/(grand total)`

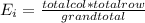
On this case we assume that the calculated statistic is given by:

Statistic calculated

`\chi^2_(calc)=13.167`

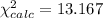
P value

Assuming the we have 2 rows and 4 columns on the contingency table.

Now we can calculate the degrees of freedom for the statistic given by:

`df=(rows-1)(cols-1)=(2-1)(4-1)=3`

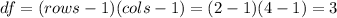
We can calculate the critical value with this formula in excel:" =CHISQ.INV(0.95,3)" On this case we got that the critical value is:

`\chi^2_(crit)=7.815`

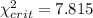
Since our calculated value is higher than the cirtical value we have enough evidence to reject the null hypothesis at the significance level of 5%.

And we can also calculate the p value given by:

`p_v = P(\chi^2_(3) >13.167)=0.0043`

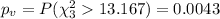
And we can find the p value using the following excel code:

"=1-CHISQ.DIST(13.167,3,TRUE)"

Since the p value is lower than the significance level we reject the null hypothesis at 5% of significance.

A. We should reject H0.

C. At the 5% significance level, there is sufficient evidence to conclude that the students were not guessing.

The reason why we select option C is because if we reject the null hypothesis of uniform distribution then we are rejecting the claim that the students are guessing.