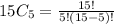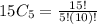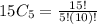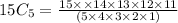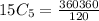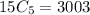Answer:
3003 number of 5-member chess teams can be chosen from 15 interested players.
Explanation:
Given:
Number of the interested players = 15
To Find:
Number of 5-member chess teams that can be chosen = ?
Solution:
Combinations are a way to calculate the total outcomes of an event where order of the outcomes does not matter. To calculate combinations, we will use the formula
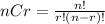
where
n represents the total number of items,
r represents the number of items being chosen at a time.
Now we have n = 15 and r = 5
Substituting the values,