Question

Consider a roulette wheel consisting of 38 numbers 1 through 36, 0, and double 0. If Smith always bets that the outcome will be one of the numbers 1 through 12,

what is the probability that
a. Smith will lose his first 5 bets;
b. his first win will occur on his fourth bet?

Answer 1

The probability that Smith will lose his first 5 bets is 0.15

The probability that his first win will occur on his fourth bet is 0.1012

Explanation:

Consider the provided information.

A roulette wheel consisting of 38 numbers 1 through 36, 0, and double 0. Smith always bets that the outcome will be one of the numbers 1 through 12,

It is given that Smith always bets on the numbers 1 through 12.

There are 12 numbers from 1 to 12.

Thus, the probability of success (winning) is=
`(12)/(38)`

The probability of not success (loses) is=
`1-(12)/(38)=(26)/(38)`

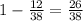
Part (A) Smith will lose his first 5 bets.

The probability that Smith loses his first 5 bets is,

`(26)/(38)*(26)/(38)*(26)/(38)*(26)/(38)*(26)/(38)=((26)/(38))^5\approx0.15`

Hence, the probability that Smith will lose his first 5 bets is 0.15

Part (B) His first win will occur on his fourth bet?

Smith’s first win occurring on the fourth bet means that he loses the first 3 bets and wins on the fourth bet. That is,

`(26)/(38)*(26)/(38)*(26)/(38)*(12)/(38)=((26)/(38))^3*(12)/(38)\approx0.1012`

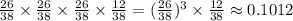
Hence, the probability that his first win will occur on his fourth bet is 0.1012