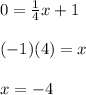Answer:

Explanation:
Remember that the line intersects the x-axis when
 .Therefore, the zero of a linear function is the value of the variable "x" when the value of "y" is zero.
.Therefore, the zero of a linear function is the value of the variable "x" when the value of "y" is zero.
The equation of the line in Slope-Intercept form is:

Where "m" is the slope and "b" is the y-intercept.
In this case, given the graph of the function,you can identify that the y-intercept is:
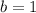
By definition, the slope can be calculated with this formula:

Then, in order to find the slope, you can pick the points (4,2) and (-8,-1) and say that:

So, substituting these values into the formula, you get:
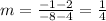
Then the linear function has this form:

Finally, in order to find the x-intercept, you can substitute
 into the function and solve for "x". This is:
into the function and solve for "x". This is: