Question

An airline’s data indicate that 50 percent of people who begin the online process of booking a flight never complete the process and pay for the flight. To reduce this percentage, the airline is considering changing its website so that the entire booking process, including flight and seat selection and payment, can be done on two simple pages rather than the current four pages. A random sample of 300 customers who begin the booking process are exposed to the new system, and 117 of them do not complete the process.

(a) Formulate the null and alternative hypotheses needed to attempt to provide evidence that the new system has reduced the noncompletion percentage.
H0: p 0.5 versus Ha: p 0.5

(b) Use critical values and a p-value to perform the hypothesis test by setting α equal to 0.10, 0.05, 0.01, and 0.001. (Negative value should be indicated by a minus sign. Round p-value to 5 decimal places and z to 2 decimal places.)
z
p-value
Since or since , there is evidence that p < 0.5.

Answer 1

a) Null hypothesis:
`p\geq 0.5`

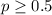
Alternative hypothesis:
`p < 0.5`

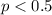
b)
`z=\frac{0.39 -0.5}{\sqrt{(0.5 (1-0.5))/(300)}}=-3.81`

`\alpha=0.1`
`z_(crit)=-1.281` excel code: "=NORM.INV(0.1,0,1)"

`\alpha=0.05`
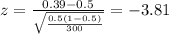
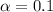
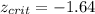`z_(crit)=-1.64` excel code: "=NORM.INV(0.05,0,1)"

`\alpha=0.01`
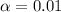
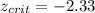`z_(crit)=-2.33` excel code: "=NORM.INV(0.01,0,1)"

`\alpha=0.001`
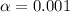
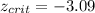`z_(crit)=-3.09` excel code: "=NORM.INV(0.001,0,1)"

For all the significance level provided we have that -3.81 <
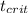`t_(crit)` so we have enough evidence to reject the null hypothesis.

`p_v =P(Z<-3.81)=1-P(z<1.34)=0.0000695`

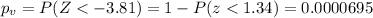
So the p value obtained was a very low value and using the significance level given
`\alpha=0.1,0.05,0.01,0.001` we have
`p_v<\alpha` so we can conclude that we have enough evidence to reject the null hypothesis, and we can said that at 5% of significance the proportion of interest is significantly less than 0.5.

Explanation:

1) Data given and notation

n=300 represent the random sample taken

X=117 represent the people with a characteristic in the sample

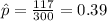`\hat p=(117)/(300)=0.39` estimated proportion of people with the characteristic desired

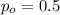`p_o=0.5` is the value that we want to test

`\alpha=0.1,0.05,0.01,0.001` represent the significance level

z would represent the statistic

`p_v` represent the p value (variable of interest)

2) Concepts and formulas to use

We need to conduct a hypothesis in order to test the claim that the population proportion is less than 0.5.:

Null hypothesis:
`p\geq 0.5`

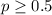
Alternative hypothesis:
`p < 0.5`

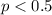
When we conduct a proportion test we need to use the z statistic, and the is given by:

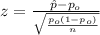`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

The One-Sample Proportion Test is used to assess whether a population proportion
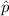`\hat p` is significantly different from a hypothesized value
`p_o`.

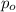3) Calculate the statistic

We can replace the values given and we got:

`z=\frac{0.39 -0.5}{\sqrt{(0.5 (1-0.5))/(300)}}=-3.81`

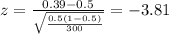
4) Statistical decision

It's important to refresh the p value method or p value approach . "This method is about determining "likely" or "unlikely" by determining the probability assuming the null hypothesis were true of observing a more extreme test statistic in the direction of the alternative hypothesis than the one observed". Or in other words is just a method to have an statistical decision to fail to reject or reject the null hypothesis.

The significance level provided are
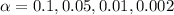`\alpha=0.1,0.05,0.01,0.002`. The next step would be calculate the p value for this test.

Since is a one right tailed test the p value would be:

`p_v =P(Z<-3.81)=1-P(z<1.34)=0.0000695`

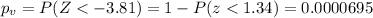
So the p value obtained was a very low value and using the significance level given
`\alpha=0.1,0.05,0.01,0.001` we have
`p_v<\alpha` so we can conclude that we have enough evidence to reject the null hypothesis, and we can said that at 5% of significance the proportion of interest is significantly less than 0.5.

5) Critical values

The critical values are given respect the significance level provided:

`\alpha=0.1`
`z_(crit)=-1.281` excel code: "=NORM.INV(0.1,0,1)"

`\alpha=0.05`
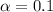
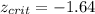`z_(crit)=-1.64` excel code: "=NORM.INV(0.05,0,1)"

`\alpha=0.01`
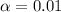
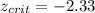`z_(crit)=-2.33` excel code: "=NORM.INV(0.01,0,1)"

`\alpha=0.001`
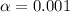
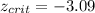`z_(crit)=-3.09` excel code: "=NORM.INV(0.001,0,1)"

For all the significance level provided we have that -3.81 <
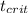`t_(crit)` so we have enough evidence to reject the null hypothesis.