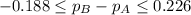Question

In a random sample of 75 American women age 18 to 30, 26 agreed with the statement that a woman should have the right to a legal abortion for any reason (question wording: "Please tell me whether or not you think it should be possible for a pregnant woman to obtain a legal abortion if the woman wants it for any reason?"). In an independent random sample of 64 American women age 58 to 70, 21 agreed with the same statement. a) Test at the 1% significance level the hypothesis that the population proportions are equal, against the alternative that the population proportions are not equal. Include the p-value of your test statistic. b) Compute a 99% confidence interval for the difference of the two age group proportions. c) Are older women more or less likely than younger women to support abortion for any reason?

Answer 1

a)
`z=\frac{0.347-0.328}{\sqrt{0.338(1-0.338)((1)/(75)+(1)/(64))}}=0.236`

`p_v =2*P(Z>0.236)=0.813`

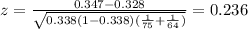
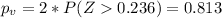
If we compare the p value and using any significance level for example
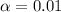`\alpha=0.01` always
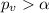`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and we can say that we don't have significant differences between the two proportions.

b) We are confident at 99% that the difference between the two proportions is between
`-0.188 \leq p_B -p_A \leq 0.226`

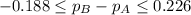
Explanation:

Previous concepts and data given

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

`p_A` represent the real population proportion of women age 18 to 30 agreed with the statement that a woman should have the right to a legal abortion for any reason

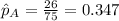`\hat p_A =(26)/(75)=0.347` represent the estimated proportion of women age 18 to 30 agreed with the statement that a woman should have the right to a legal abortion for any reason

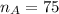`n_A=75` is the sample size for A

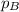`p_B` represent the real population proportion for women age 58 to 70 agreed with the statement that a woman should have the right to a legal abortion for any reason

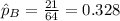`\hat p_B =(21)/(64)=0.328` represent the estimated proportion of women age 58 to 70 agreed with the statement that a woman should have the right to a legal abortion for any reason

`n_B=64` is the sample size required for B

`z` represent the critical value for the margin of error and for the statisitc

The population proportion have the following distribution

`p \sim N(p,\sqrt{(p(1-p))/(n)})`

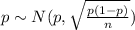
Part a

We need to conduct a hypothesis in order to check if the proportion are equal, the system of hypothesis would be:

Null hypothesis:
`p_(A) = p_(B)`

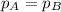
Alternative hypothesis:
`p_(A) \\eq p_(B)`

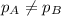
We need to apply a z test to compare proportions, and the statistic is given by:

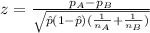`z=\frac{p_(A)-p_(B)}{\sqrt{\hat p (1-\hat p)((1)/(n_(A))+(1)/(n_(B)))}}` (1)

Where
`\hat p=(X_(A)+X_(B))/(n_(A)+n_(B))=(26+21)/(75+64)=0.338`

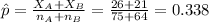
Calculate the statistic

Replacing in formula (1) the values obtained we got this:

`z=\frac{0.347-0.328}{\sqrt{0.338(1-0.338)((1)/(75)+(1)/(64))}}=0.236`

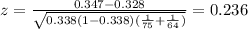
Statistical decision

Since is a two sided test the p value would be:

`p_v =2*P(Z>0.236)=0.813`

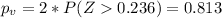
If we compare the p value and using any significance level for example
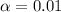`\alpha=0.01` always
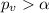`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and we can say that we don't have significant differences between the two proportions.

Part b

The confidence interval for the difference of two proportions would be given by this formula

`(\hat p_A -\hat p_B) \pm z_(\alpha/2) \sqrt{(\hat p_A(1-\hat p_A))/(n_A) +(\hat p_B (1-\hat p_B))/(n_B)}`

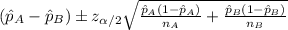
For the 99% confidence interval the value of
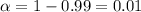`\alpha=1-0.99=0.01` and
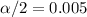`\alpha/2=0.005`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=2.58`

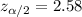
And replacing into the confidence interval formula we got:

`(0.347-0.328) - 2.58 \sqrt{(0.347(1-0.347))/(75) +(0.328(1-0.328))/(64)}=-0.188`

`(0.347-0.328) + 2.58 \sqrt{(0.347(1-0.347))/(75) +(0.328(1-0.328))/(64)}=0.226`

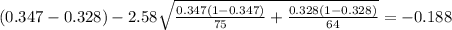
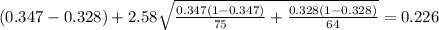
And the 99% confidence interval for the difference of proportions would be given (-0.188;0.226).

We are confident at 99% that the difference between the two proportions is between
`-0.188 \leq p_B -p_A \leq 0.226`