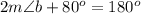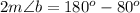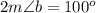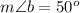Answer:
Part 1) Option A.
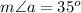
Part 2) Option B.
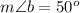
Explanation:
we know that
An isosceles triangle has two equal sides and two equal angles
Part 1) what is the measure of ∠a?
we know that
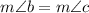 ----> equation A
----> equation A
Remember that the sum of the interior angles of triangle is equal to 180 degrees
we have
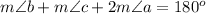 ----> equation B
----> equation B
substitute equation A in equation B
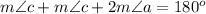
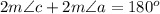
Divide by 2 both sides
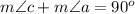
we have
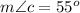 ---> given problem
---> given problem
substitute
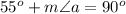
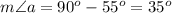
Part b) we have that
we have
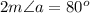 ---> given problem
---> given problem
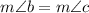 ---> equation A
---> equation A
Remember that the sum of the interior angles of triangle is equal to 180 degrees
we have
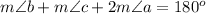 ----> equation B
----> equation B
substitute equation A in equation B
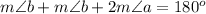
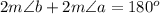
we have
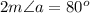
substitute