Question

Phosgene, COCl2, gained notoriety as a chemical weapon in World War I. Phosgene is produced by the reaction of carbon monoxide with chlorine, CO(g) Cl2(g) <-------> COCl2(g) The value of Kc for this reaction is 5.79 at 570 K. What are the equilibrium partial pressures of the three gases if a reaction vessel initially contains a mixture of the reactants in which PCO?

Answer 1

The equilibrium partial pressures of CO, Cl2, and COCl2 are 5.79 atm, 5.79 atm, and 0.10 atm, respectively.

Step-by-step explanation:

The equilibrium partial pressures of the three gases can be determined using the equilibrium expression and the given information. The equilibrium expression for the phosgene-forming reaction is:

PCOCl2 = (PCO * PCl2) / PCOCl2,eq

Given that the equilibrium constant (Kc) is 5.79, the equilibrium partial pressures can be calculated as follows:

PCO = (PCOCl2,eq / PCl2) * Kc

PCl2 = (PCOCl2,eq / PCO) * Kc

Substituting the given information:

PCO = (0.10 atm / 0.10 atm) * 5.79 = 5.79 atm

PCl2 = (0.10 atm / 0.10 atm) * 5.79 = 5.79 atm

PCOCl2,eq = 0.10 atm

The equilibrium partial pressures of CO, Cl2, and COCl2 are 5.79 atm, 5.79 atm, and 0.10 atm, respectively.

Answer 2

The question is incomplete, here is the complete question:

Phosgene,
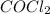`COCl_2`, gained notoriety as a chemical weapon in World War I. Phosgene is produced by the reaction of carbon monoxide with chlorine:

`CO(g)+Cl_2(g)\rightleftharpoons COCl_2(g)`

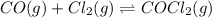
The value of
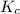`K_c` for this reaction is 5.79 at 570 K. What are the equilibrium partial pressures of the three gases if a reaction vessel initially contains a mixture of the reactants in which
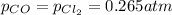`p_(CO)=p_(Cl_2)=0.265atm` and
`p_(COCl_2)=0.000atm` ?

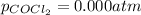Answer: The equilibrium partial pressure of CO,
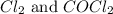`Cl_2\text{ and }COCl_2` is 0.257 atm, 0.257 atm and 0.008 atm respectively.

Step-by-step explanation:

The relation of
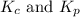`K_c\text{ and }K_p` is given by:

`K_p=K_c(RT)^(\Delta n_g)`

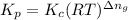
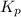`K_p` = Equilibrium constant in terms of partial pressure

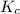`K_c` = Equilibrium constant in terms of concentration = 5.79

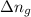`\Delta n_g` = Difference between gaseous moles on product side and reactant side =
`n_(g,p)-n_(g,r)=1-2=-1`

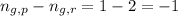
R = Gas constant =
`0.0821\text{ L. atm }mol^(-1)K^(-1)`

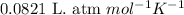
T = Temperature = 570 K

Putting values in above equation, we get:

`K_p=5.79* (0.0821* 570)^(-1)\\\\K_p=0.124`

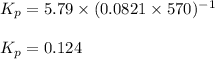
We are given:

Initial partial pressure of CO = 0.265 atm

Initial partial pressure of chlorine gas = 0.265 atm

Initial partial pressure of phosgene = 0.00 atm

The given chemical equation follows:

`CO(g)+Cl_2(g)\rightleftharpoons COCl_2(g)`

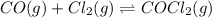
Initial: 0.265 0.265

At eqllm: 0.265-x 0.265-x x

The expression of
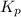`K_p` for above equation follows:

`K_p=(p_(COCl_2))/(p_(CO)* p_(Cl_2))`

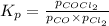
Putting values in above equation, we get:

`0.124=(x)/((0.265-x)* (0.265-x))\\\\x=0.0082,8.59`

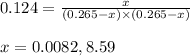
Neglecting the value of x = 8.59 because equilibrium partial pressure cannot be greater than initial pressure

So, the equilibrium partial pressure of CO =
`(0.265-x)=(0.265-0.008)=0.257atm`

The equilibrium partial pressure of
`Cl_2=(0.265-x)=(0.265-0.008)=0.257atm`

The equilibrium partial pressure of
`COCl_2=x=0.008atm`

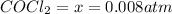
Hence, the equilibrium partial pressure of CO,
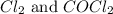`Cl_2\text{ and }COCl_2` is 0.257 atm, 0.257 atm and 0.008 atm respectively.