Question

For atomic hydrogen, the Paschen series of lines occurs when nf = 3, whereas the Brackett series occurs when nf = 4 in the equation

1/?= 2p2 mk2 e4/ h3c (Z2) (1/n2f - 1/n2i)

Using this equation, show that the ranges of wavelengths in these two series overlap.
Shortest Wavelength (m) Longest Wavelength (m)

Paschen Series

Answer 1

The Paschen and Brackett series of lines in atomic hydrogen can overlap in terms of their wavelength ranges.

Step-by-step explanation:

The Paschen series of lines in atomic hydrogen occurs when nf = 3, and the Brackett series occurs when nf = 4.

The range of wavelengths in these two series can overlap because the wavelength equation 1/λ = 2π²mk²e⁴/(h³c)(Z²)(1/nf² - 1/ni²) depends on the values of nf and ni, which can be different for each series

For example, if nf = 3 in the Paschen series and nf = 4 in the Brackett series, the wavelengths in these two series can overlap depending on the values of ni.

Answer 2

the highest value of the Paschen series is 18805 μm which is greater than the shortest value of the Brackett series (14617 μm).

Step-by-step explanation:

Hydrogen atom transitions are described by the Bohr model

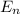`E_(n)` = -13.606 / n²

Where n is an integer

Transitions occur between two states with different quantum numbers n

ΔE = -13.606 (1 /
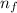`n_(f)`² - 1 /
`n_(i)`²)

Where
`n_(f)` >
`n_(i)`

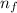For the wavelength we use the Planck equation

E = h f

c = λ f

E = h c / λ

λ = h c / E

λ = 6.63 10⁻³⁴ 3 10⁸ / E

λ = 19.89 10⁻²⁶ / E

Let's reduce to eV

λ = 19.89 10⁻²⁶ / E[J] (Ev / 1.6 10⁻¹⁹ J)

Lam = 12430 / E [eV]

Let's calculate the energy for transitions

Paschen Series

`n_(f)`
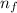
`n_(i)` ΔE (eV) λ (um)

4 3 0.661 18805

5 3 0.9657 12871

10 3 1.3757 9035

100 3 1.5104 8229

∞ 3 1.5117 8222

Brackett Series

`n_(f)`
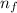
`n_(i)` ΔE (eV) λ (um)

5 4 0.3061 40607

6 4 0.4724 26312

10 4 0.7143 17401

100 4 0.8490 14640

∞ 4 0.8504 14617

When we analyze the wavelength values ​​we see that the highest value of the Paschen series is 18805 μm which is greater than the shortest value of the Brackett series (14617 μm).