Answer:
The answer to your question is letter C
Explanation:
Process
1.- Find two points of the dotted line
A (0, 4)
B (3, 5)
2.- Find the slope of the line
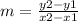
Substitution
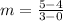
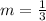
3.- Write the equation of the line
y - y1 = m(x - x1)
y - 4 = 1/3(x - 0)
y - 4 = 1/3x
y = 1/3x + 4
4.- Write the inequality
We are interested on the upper area so
y > 1/3x + 4