Question

There are 22 4th grade students and 26 fifth grade students who became cheerleaders each cheer leading group will have 8 members what equation can be used to find out how many groups can be made

Answer 1

The equation to find the number of groups can be formed is given as:
`n=(22+26)/(8)`

where
`n` represent the number of groups that can be formed.

We get
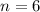`n=6` on solving

Explanation:

Number of 4th grade students who become cheerleader = 22

Number of 5th grade students who become cheerleader = 26

Number of cheerleaders in each cheer leading groups = 8

We need to divide the total number of cheer leaders into groups of 8.

Let
`n` represent the number of groups that can be formed.

Total number of students who want to become cheerleaders =
`22+26`

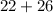
So, equation to find the number of groups can be given as :

`n=(22+26)/(8)`

⇒
`n=(48)/(8)`

⇒
`n=6`

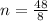
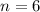
Thus,a total of 6 cheer leading groups can be formed.