Question

Does (1, 1) make the inequality 2x + 17y ≥ 19 true?

plezzzzzzzzzzzzz help

Answer 1

The point (1, 1) makes the inequality
`2x+17y\geq 19` true.

Explanation:

Given inequality:

`2x+17y\geq 19`

Point (1,1)

To check if the inequality is true for the given point.

Solution:

For a point
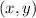`(x,y)` to make the inequality true it must be a solution of the inequality.

To check if point (1,1) lies within the inequality range, we will plugin the
`x` and
`y` values of the point in the given inequality.

Plugging in
`x=1` and
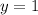`y=1` in the inequality.

`2(1)+17(1)\geq 19`

`2+17\geq 19`

`19\geq 19`

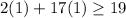
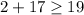
Thus it satisfies the inequality as 19 is included in the inequality.

The graph of the inequality
`2x+17y\geq 19` and the location of the point (1,1) is shown below.

From the graph, we can see that (1,1) lies on the line which is included in the solution of the inequality.

Thus, we say point (1, 1) makes the inequality
`2x+17y\geq 19` true.