Question

The half life for the decay of carbon-14 is 5.73 x 10^3 years. Suppose the activity due to the radioactive decay of the carbon-14 in a tiny sample of an artifact made of wood from an archeological dig is measured to be 53.Bq. The activity in a similar-sized sample of fresh wood is measured to be 59.Bq.

1. Calculate the age of the artifact. Round your answer to 2 significant digits.

Answer 1

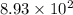`8.93* 10^2` years

Step-by-step explanation:

Given that:

Half life = 5730 years

`t_(1/2)=\frac {ln\ 2}{k}`

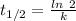
Where, k is rate constant

So,

`k=\frac {ln\ 2}{t_(1/2)}`

`k=(ln\ 2)/(5730)\ years^(-1)`

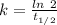
The rate constant, k = 0.00012 years⁻¹

Initial concentration [A₀] = 59 Bq

Final concentration
`[A_t]` = 53 Bq

Time = ?

Using integrated rate law for first order kinetics as:

`[A_t]=[A_0]e^(-kt)`

Applying values, we get that:-

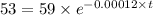
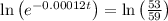
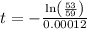
`53=59* e^(-0.00012* t)`

`\ln \left(e^(-0.00012t)\right)=\ln \left((53)/(59)\right)`

`t=-(\ln \left((53)/(59)\right))/(0.00012)`

t =
`8.93* 10^2` years

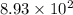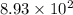`8.93* 10^2` years is the age of the artifact.