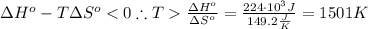Question

The standard reaction enthalpy of Zn(s) + H2O(g) →ZnO(s) + H2(g) is known to be HR 0 = 224 kJ and is approximately constant from 920 K up to 1280 K. The standard reaction free energy is +33 kJ at 1280 K. Calculate the equilibrium constant at 1280 K and then calculate the temperature at which the equilibrium constant becomes greater than 1.

Answer 1

K = 0.0450, T > 1501 K

Step-by-step explanation:

We may apply the Gibbs free energy equation relating the Gibbs free energy to an equilibrium constant of a reaction. The relationship is described by the following equation:

`\Delta G^o = -RT ln(K)`

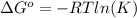
Rearrange the equation for the equilibrium constant:

`ln(K) = -(\Delta G^o)/(RT)\therefore K = e^{-(\Delta G^o)/(RT)}`

Given the temperature
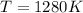`T = 1280 K` and the ideal gas law constant
`R = 8.314 (J)/(K mol)`, we obtain:

`K = e^{-(33\cdot 10^3 J)/(8.314 (J)/(K mol)\cdot 1280 K)} = 0.0450`

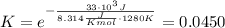
Now notice if
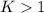`K > 1`, then
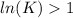`ln(K) > 1` and
`\Delta G^o < 0`.

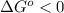We may firstly solve for the entropy change of this reaction using the following equation:

`\Delta G^o = \Delta H^o - T\Delta S^o \therefore \Delta S^o = (\Delta H^o - \Delta G^o)/(T) = (224\cdot 10^3 J - 33\cdot 10^3 J)/(1280 K) = 149.2 (J)/(K)`

Using the same equation, solve when the change in the Gibbs free energy is negative:

`\Delta H^o - T\Delta S^o < 0\therefore T > (\Delta H^o)/(\Delta S^o) = (224\cdot 10^3 J)/(149.2 (J)/(K)) = 1501 K`