Answer:
The horse is going at 12.72 m/s speed.
Step-by-step explanation:
The initial speed of the horse (u) = 3 m/s
The acceleration of the horse (a)= 5 m/

The displacement( it is assumed it is moving in a straight line)(s)= 15.3 m
Applying the second equation of motion to find out the time,
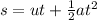
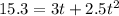
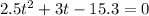
Solving this quadratic equation, we get time(t)=1.945 s, the other negative time is neglected.
Now applying first equation of motion, to find out the final velocity,

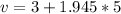
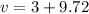
v=12.72 m/s
The horse travels at a speed of 12.72 m/s after covering the given distance.