Answer:
So the correct option is C ) 58 units.
Also If we require Distance then l(AC) = √58 = 7.615 units
Explanation:
Let the Points be
point A( x₁ , y₁) ≡ ( -2 , 1)
point C( x₂ , y₂) ≡ (5 , -2)
To Find:
d(AC) = ?
Solution:
By Applying the Pythagorean Theorem to find the distance between points A and C we get
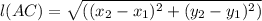
Substituting A( x₁ , y₁) ≡ ( -2 , 1) and C( x₂ , y₂) ≡ (5 , -2) we get
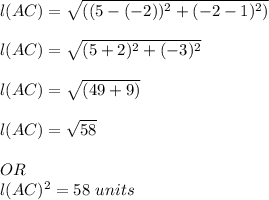
So the correct option is C ) 58 units.
If we require Distance then l(AC) = √58 = 7.615 units