Question

Which group represents equivalent ratios?

Group of answer choices

75%, 2/4, 0.8

70%, 7/10, 0.7

75%, 7/10, 0.25

70%, 1/7, 0.7

Answer 1

The group
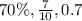`70 \%, (7)/(10), 0.7` represents equivalent ratios.

Answer: Option B

Explanation:

As we see carefully, the equation B represents the equivalent ratio. Because if you divide the 7 by 10 then you will get 0.7 and this 0.7 can also be written as 70% in percentage form. So, the correct answer is Option B.

Case A:
`75 \%, (2)/(4), 0.8`

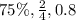
It can be written as that 0.75, 0.5, 0.8.this group does not represent equivalent ratios

Case B:
`70 \%, (7)/(10), 0.7`

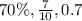
If you divide the 7 by 10 then you will get 0.7 and this 0.7 can also be written as 70% in percentage form. This group represents equivalent ratios

Case C:
`70 \%, (7)/(10), 0.25`

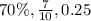
It can writes as that 0.7,0.7.0.25 so this group does not represent equivalent ratios

Case D:
`70 \%, (1)/(7), 0.7`

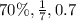
This gives 0.7, 0.14, 0.7 so this group does not represent equivalent ratios.