Answer:
(a)
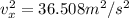
(b) 0.1235 Pa
(c) The volume of the ball is significant when compared with the volume of the closed room. Thus, the constant 'b' of the equation of Van der Waal is greater than zero.
Step-by-step explanation:
In the question, we are given the following variables:
V = 453 m^3
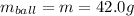
Kinetic energy (K.E) = 2.30 J
(a) The equation for kinetic energy is:
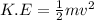
Since the average velocity components of the ball in the three dimensions are equal. Thus, we have:
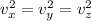
In addition:
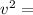
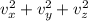 =
=
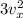
Therefore:
![K.E = (1)/(2)m[3v^(2) _(x)]](https://img.qammunity.org/2020/formulas/physics/high-school/izioy6qr37rsui91fw129zayaqkm1267l7.png) =
=

Thus:
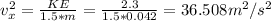
It does not matter which direction we take as x-direction because the average velocity component in every direction is the same.
(b) The average pressure on the walls can be calculated using the equation below:
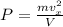
where V = 453 m^3
Thus: P = (0.042*36.508^2)/453 = 0.1235 Pa
(c) The volume of the ball is significant when compared with the volume of the closed room. Thus, the constant 'b' of the equation of van der Waal is greater than zero.