Question

Calculate "de Broglie" wavelength for each of the following, and use your numerical answers the to explain why macroscopic (large) objects are not ordinarily discussed in terms of their "wave-like" properties. a. an electron moving at .90 times the speed of light.

b. a 150-g ball moving at a speed of 10.m/s​

Answer 1

a.
`2.69 pm`

b.
`4.42\cdot 10^(-34) m`

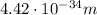
Step-by-step explanation:

The de Broglie wavelength can be found by the following equation:

`\lambda_(dB) = (h)/(mv)`

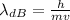
Here:

`\lambda_(dB)` is the de Broglie wavelength (in m);

`h` is the Planck's constant,
`h = 6.626\cdot 10^(-34) J\cdot s`;

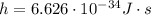`m` is mass (in kg);

`v[tex] is velocity (in m/s).</p><p>a. We need to know the mass of an electron here:</p><p>[tex]m_e=9.11\cdot10^(-31) kg`

And the speed of light:

`c = 3.00\cdot 10^8 m/s`

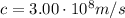
The fraction of the speed of light is:

`\omega = 0.90`

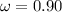
Substituting into the equation:

`\lambda_(dB) = (h)/(\omega c m_e)=(6.626\cdot10^(-34) J\cdot s)/(0.90\cdot 9.11\cdot 10^(-31) kg\cdot 3.00\cdot 10^8 m/s) = 2.69\cdot 10^(-12) m = 2.69 pm`

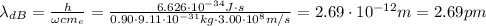
b. Similarly, here we have:

`m_b=150 g = 0.150 kg`

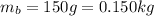
And the velocity of:

`v = 10 m/s`

We obtain:

`\lambda_(dB)={6.626\cdot 10^(-34) J\cdot s}{0.150 kg\cdot 10 m/s} = 4.42\cdot 10^(-34) m`

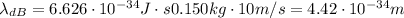
Notice that the wavelength of a large object is smaller by a fraction of:

`(2.69\cdot 10^(-12) m)/(4.42\cdot 10^(-34) m) = 6\cdot 10^(21)`

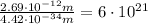
This means the de Broglie wavelength of a macroscopic object is negligible compared to the wavelength of a microscopic object.