Question

Abby bought two slices of pizza and

three bottles of water for $7.25
Cameron bought four slices of pizza and
one bottle of water for $8.25 what is the
solution

Answer 1

The solution is price of 1 slice of pizza is $ 1.75 and price of 1 bottle of water is $ 1.25

Solution:

Let "p" be the price of 1 slice of pizza

Let "b" be the price of 1 bottle of water

Given that Abby bought two slices of pizza and three bottles of water for $7.25

So we can frame a equation as:

two slices of pizza x price of 1 slice of pizza + three bottles of water x price of 1 bottle of water = $ 7.25

`2 * p + 3 * b = 7.25`

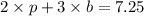
2p + 3b = 7.25 ------- eqn 1

Cameron bought four slices of pizza and one bottle of water for $8.25

So we can frame a equation as:

four slices of pizza x price of 1 slice of pizza + one bottles of water x price of 1 bottle of water = $ 8.25

`4 * p + 1 * b = 8.25`

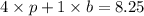
4p + 1b = 8.25 ----- eqn 2

Let us solve eqn 1 and eqn 2 to find values of "p" and "b"

Multiply eqn 1 by 2

4p + 6b = 14.5 ----- eqn 3

Subtract eqn 2 from eqn 3

4p + 6b = 14.5

4p + 1b = 8.25

( - ) ------------------

5b = 6.25

b = 1.25

Substitute b = 1.25 in eqn 1

2p + 3b = 7.25

2p + 3(1.25) = 7.25

2p + 3.75 = 7.25

2p = 3.5

p = 1.75

Thus the solution is:

price of 1 slice of pizza = $ 1.75

price of 1 bottle of water = $ 1.25