Answer:
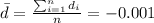

-The sample is too small to make judgments about skewness or symmetry.
H0:
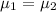
H1:
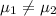
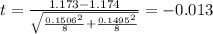
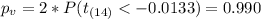
So the p value is a very high value and using any significance level for example
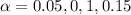 always
always
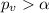 so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and a we don't have a significant difference between the two means.
so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and a we don't have a significant difference between the two means.
Explanation:
First we need to find the difference defined as:
(Operator 1 minus Operator 2)
d1=1.326-1.323=0.003 d2=1.337-1.322=0.015
d3=1.079-1.073=0.006 d4=1.229-1.233=-0.004
d5=0.936-0.934=0.002 d6=1.009-1.019=-0.01
d7=1.179-1.184=-0.005 d8=1.289-1.304=-0.015
Now we can calculate the mean of differences given by:
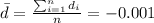
And for the sample deviation we can use the following formula:

Describe the distribution of these differences using words. (which one is correct)
We can plot the distribution of the differences with the folowing code in R
differences<-c(0.003,0.015,0.006,-0.004,0.002,-0.01,-0.005,-0.015)
hist(differences)
And we got the image attached. And we can see that the distribution is right skewed but we don't have anough info to provide a conclusion with just 8 differnences.
-The sample is too small to make judgments about skewness or symmetry.
Use a significance test to examine the null hypothesis that the two operators have the same mean. Give the test statistic. (Round your answer to three decimal places.)
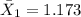 represent the mean for the operator 1
represent the mean for the operator 1
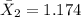 represent the mean for the operator 2
represent the mean for the operator 2
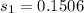 represent the sample standard deviation for the operator 1
represent the sample standard deviation for the operator 1
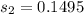 represent the sample standard deviation for the operator 2
represent the sample standard deviation for the operator 2
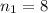 sample size for the operator 1
sample size for the operator 1
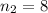 sample size for the operator 2
sample size for the operator 2
t would represent the statistic (variable of interest)
Concepts and formulas to use
We need to conduct a hypothesis in order to check if the means for the two groups are the same, the system of hypothesis would be:
H0:
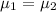
H1:
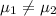
If we analyze the size for the samples both are less than 30 so for this case is better apply a t test to compare means, and the statistic is given by:
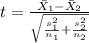 (1)
(1)
t-test: Is used to compare group means. Is one of the most common tests and is used to determine whether the means of two groups are equal to each other.
Calculate the statistic
We can replace in formula (1) like this:
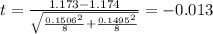
Statistical decision
For this case we don't have a significance level provided
 , but we can calculate the p value for this test. The first step is calculate the degrees of freedom, on this case:
, but we can calculate the p value for this test. The first step is calculate the degrees of freedom, on this case:
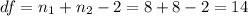
Since is a bilateral test the p value would be:
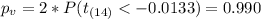
So the p value is a very high value and using any significance level for example
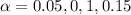 always
always
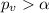 so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and a we don't have a significant difference between the two means.
so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and a we don't have a significant difference between the two means.