Answer:
a) (M / m -1), b)
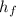 = h (M / m -1)²
= h (M / m -1)²
Step-by-step explanation:
For this exercise we will begin by looking for the speed of the balls when they reach the floor, for this we use the concepts of energy conservation
Initial. Highest point
Em₀ = U = m g h
Final. Floor
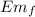 = K = ½ m v²
= K = ½ m v²
Em₀ =
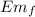
m gh = ½ m v²
v = √ 2g h
The speed is the same for the two balls since it does not depend on the mass.
First shock
The heaviest ball (M) with the floor, as the floor does not move the ball bounces with the same speed as it arrives, but in the opposite direction
Second shock
Between the large ball with velocity upwards of value v and the small ball of mass (m) with velocity v downwards. Let's use the moment
Initial. Before the crash
p₀ = Mv - m v
After the crash
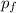 = 0 + m
= 0 + m

p₀ =
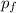
Mv - m v = m

 = (M-m) / m v
= (M-m) / m v
 = (M / m -1) v
= (M / m -1) v
Let's look with energy to where e raises the small ball with speed vf
Em₀ =

½ m vf² = m g
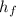
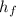 = ½
= ½
 ² / g
² / g
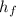 = ½ v² / g (M / m-1)²
= ½ v² / g (M / m-1)²
We substitute in value of v
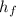 = ½ 2gh / g (M / m -1)²
= ½ 2gh / g (M / m -1)²
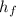 = h (M / m -1)²
= h (M / m -1)²