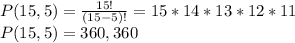Answer:
1) 0.5 or 50%.
2) Order does not matter: 3,003 combinations.
Order matters: 360,360 permutations.
Explanation:
1) The probability of drawing either a purple or a blue chip is the sum of both individual probabilities:
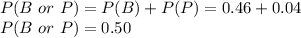
the probability that a blue or a purple chip will be drawn is 0.5 or 50%.
2)
a) Choose 5 items from a total of 15 items when order does not matter:
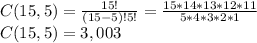
b) Choose 5 items from a total of 15 items when order matters: