Question

A bank with branches located in a commercial district of a city and in a residential district has the business

objective of developing an improved process for serving customers during the noon-to-1 P.M. lunch
period. Management decides to first study the waiting time in the current process. The waiting time is
defined as the time that elapses from when the customer enters the line until he or she reaches the teller
window. Data are collected from a random sample of 15 customers at each branch.

The following is the data sample of the wait times, in minutes, from the commercial district branch.

4.14 5.66 3.04 5.34 4.82 2.69 3.32 3.41
4.42 6.01 0.15 5.11 6.59 6.43 3.72

The following is the data sample of the wait times, in minutes, from the residential district branch.

9.99 5.89 8.06 5.91 8.64 3.77 8.21 8.52
10.46 6.87 5.53 4.23 6.25 9.88 5.59

Determine the test statistic.

Answer 1

test statistic is 4.27

Explanation:

`H_(0)` : mean waiting time in a residential district branch is the same as a commercial district branch

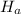`H_(a)` : mean waiting time in a residential district branch is more than a commercial district branch

commercial district branch:

mean waiting time:
`(4.14+5.66+3.04+5.34+4.82+2.69+3.32+3.41+4.42+6.01+0.15+5.11+6.59+6.43+3.72)/(15) =4.32`

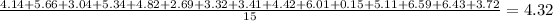
standard deviation:

mean squared differences from the mean = 1.63

residential district branch.

mean waiting time:
`(9.99+5.89+8.06+5.91+8.64+3.77+8.21+8.52+10.46+6.87+5.53+4.23+6.25+9.88+5.59)/(15) =7.19`

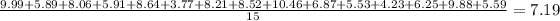
standard deviation:

mean squared differences from the mean = 2.03

test statstic can be calculated using the formula:

`z=\frac{X-Y}{\sqrt{(s(x)^2)/(N(x))+(s(y)^2)/(N(y))}}` where

• X is the mean mean waiting time for residential district branch. (7.19)

• Y is the mean mean waiting time for commercial district branch. (4.32)

• s(x) is the sample standard deviation for residential district branch (2.03)

• s(y) is the sample standard deviation for commercial district branch.(1.93)

• N(x) is the sample size for residential district branch (15)

• N(y) is the sample size for commercial district branch.(15)

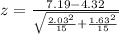`z=\frac{7.19-4.32}{\sqrt{(2.03^2)/(15)+(1.63^2)/(15)}}` ≈4.27