Question

What is the poinet-slope form of the equation for the line in the graph?​

Answer 1

`y -5 = (9)/(13)(x - 7)`

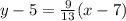
Explanation:

Given :

Two points are given in graph (-6, -4) and {7, 5).

The point-slope form of the equation of a straight line is:

`y -y_(1) = m(x - x_(1))`------------(1)

Let
`(x_(1), y_(1))=(7,5)` and
`(x_(2), y_(2))=(-6,-4)`

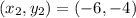
The slope of the line
`m=(y_(2)- y_(1))/(x_(2)- x_(1))`

Put all known value in above equation.

`m=(-4- 5)/(-6- 7)`

`m=(-9)/(-13)`

`m=(9)/(13)`

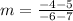
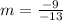
The slope of the line
`m=(9)/(13)`

We know m, and also know that
`(x_(1), y_(1))=(7,5)`, so we put these value in equation 1.

`y -5 = (9)/(13)(x - 7)`

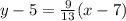
Therefore, the equation of the line is
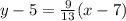`y -5 = (9)/(13)(x - 7)`.