Question

Question 8 of

Question 8 (2 points)
What values of x satisfy the equation 2x2 + 3x – 10 = 2x + 5
a
x
=3/2 or x=-5
x=-5/2 or x=2
x=10 or x=-1/2
X=-3 or x=5/2
Next Page
Back

Answer 1

`x=-3\ or\ (5)/(2)`

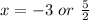
Explanation:

Given:

Given equation is.

`2x^(2) +3x-10=2x+5`

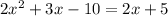
Find values of x?

Solution.

`2x^(2) +3x-10=2x+5`

`2x^(2) +3x-10-2x-5=0`

`2x^(2) +x-15=0`

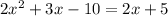
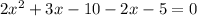
Find the roots of the equation.

compare the above equation with
`ax^(2) +bx+c=0`

Therefore,
`a=2,b=1,c=-15`

`x=\frac{-b\pm\sqrt{(b)^(2)-4ac}}{2a}`

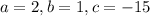
Put a,b and c value in above equation.

`x=\frac{-1\pm\sqrt{(1)^(2)-4(2)(-15)}}{2(2)}`

`x=(-1\pm√(1-8(-15)))/(4)`

`x=(-1\pm√(1+120))/(4)`

`x=(-1\pm√(121))/(4)`

`x=\frac{-1\pm\sqrt{(11)^(2)}}{4}`

`x=(-1\pm 11)/(4)`

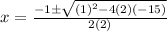
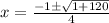
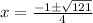
For positive sign

`x=(-1+ 11)/(4)`

`x=(10)/(4)`

`x=(5)/(2)`

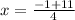
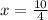
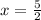
For negative sign

`x=(-1- 11)/(4)`

`x=(-12)/(4)`

`x=-3`

`x=-3\ or\ (5)/(2)`

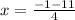
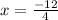
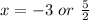
Therefore, the value of
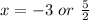`x=-3\ or\ (5)/(2)` satisfy the given equation.