Question

Ten narrow slits are equally spaced 2.00 mm apart and illuminated with green light of wavelength 511 nm. The width of bright fringes can be calculated as the separation between the two adjacent dark fringes on either side. Find the angular widths (in rad) of the third- and fifth-order bright fringes. third-order bright fringe rad fifth-order bright fringe rad

Answer 1

The width of third and fifth order bright fringe is 0.00076 rad and 0.00127 rad.

Step-by-step explanation:

Given that,

Distance d = 2.00 mm

Wavelength = 511 nm

Order number = 3

Order number = 5

We need to calculate the width of third-order bright fringe

Using formula of width

`d\sin\theta=m\lambda`

`\theta=\sin^(-1)(m\lambda)/(d)`

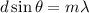
Put the value into the formula

`\theta=\sin^(-1)(3*511*10^(-9))/(2.00*10^(-3))`

`\theta=0.00076\ rad`

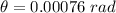
We need to calculate the width of fifth-order bright fringe

Using formula of width

`d\sin\theta=m\lambda`

`\theta=\sin^(-1)(m\lambda)/(d)`

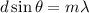
Put the value into the formula

`\theta=\sin^(-1)(5*511*10^(-9))/(2.00*10^(-3))`

`\theta=0.00127\ rad`

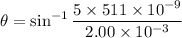
Hence, The width of third and fifth order bright fringe is 0.00076 rad and 0.00127 rad.