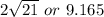Find the diagonal(d) of the regular solid.

Question

asked Jun 11, 2020 48.4k views

1 Answer

Themilkyninja · Answer 1 · 2020-06-16T08:30:16+0000

Answer:

The diagonal of the given regular solid is
$2√(21)\ or\ 9.165$

Explanation:

Given:

The given regular solid is a cuboid or a rectangular prism.

Length of the cuboid is,
l=8

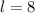
Width of the cuboid is,
w=4

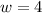
Height of the cuboid is,
h=2

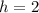
We know that, for a cuboid of length 'l', width 'w' and height 'h', the diagonal length 'd' is given as:

d=√(l^2+w^2+h^2)

Plug in 8 for 'l', 4 for 'w', 2 for 'h' and solve for 'd'. This gives,

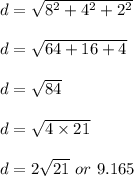
$d=√(8^2+4^2+2^2)\\\\d=√(64+16+4)\\\\d=√(84)\\\\d=√(4* 21)\\\\d=2√(21)\ or\ 9.165$

Therefore, the diagonal of the given regular solid is
$2√(21)\ or\ 9.165$