Question

The moment of inertia of a thin ring of mass M and radius R about its symmetry axis is ICM = MR2.

What is the moment of inertia of a large ring if you twirl it around your finger, so that in essence it rotates about a point on the ring, about an axis parallel to the symmetry axis?
A)2MR^2
B).5MR^2
C)1.5MR^2
D)5MR^2
E)MR^2

Answer 1

The moment of inertia of large ring is 2MR².

(A) is correct option.

Step-by-step explanation:

Given that,

Mass of ring = M

Radius of ring = R

Moment of inertia of a thin ring = MR²

Moment of inertia :

Moment of inertia is the product of the mass of the ring and square of radius of the ring.

We need to calculate the moment of inertia of large ring

Using formula of moment of inertia

`I=I_(cm)+MR^2`

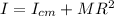
Where,
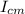`I_(cm)` = moment of inertia at center of mass

M = mass of ring

R = radius of ring

Put the value into the formula

`I=MR^2+MR^2`

`I=2MR^2`

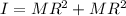
Hence, The moment of inertia of large ring is 2MR².