Question

One leg of a 45 - 45 - 90 triangle measures 10 inches. What is the length of the hypotenuse

Answer 1

Therefore the length of the hypotenuse is 14.14 inches.

Explanation:

Given:

The Triangle is of 45 -45 - 90

Length of one leg = 10 inches

To Find:

Length of the hypotenuse = ?

Solution:

The Triangle is of 45 -45 - 90 ..................Given

So For 45 - 45 - 90 Both the Legs of Triangle is SAME

i.e Longer leg = Shorter leg = 10 inches

Now in Right angle Triangle By Pythagoras Theorem we have

`(\textrm{Hypotenuse})^(2) = (\textrm{Shorter leg})^(2)+(\textrm{Longer leg})^(2)`

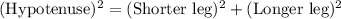
On substituting the values we get

`Hypotenuse^(2)= 10^(2)+ 10^(2) \\\\Hypotenuse^(2)=200\\Square\ Rooting\ we\ get\\Hypotenuse=√(200)=10√(2)=10* 1.414=14.14\ inches`

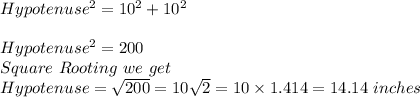
Therefore the length of the hypotenuse is 14.14 inches.