Answer:
Induced emf,
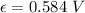
Step-by-step explanation:
It is given that,
Radius of the circular loop, r = 10.9 cm = 0.109 m
Magnetic field, B = 0.797 T
When released, the radius of the loop starts to shrink at an instantaneous rate of 107 cm/s,
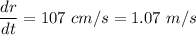
Due to change in loop of the loop, an emf is induced in the loop. It is given by :
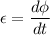
 = magnetic flux
= magnetic flux
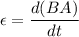
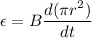
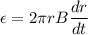
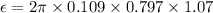
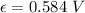
So, the emf is induced in the loop at that instant is 0.584 volts. Hence, this is the required solution.