Answer:
a) I =
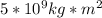
b) L =
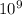 (kg*m^2)/s
(kg*m^2)/s
c)
 = 233.3m/s
= 233.3m/s
d)
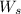 = 0.1 rad/s
= 0.1 rad/s
Step-by-step explanation:
a) We know that:
I=
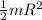
where I is the moment of inertia, m the mass and R the radius. So, replacing values, we get:
I=

I =
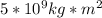
b) We know that:
L = IW
where L is the angular momentum, I the moment of inertia and W the angular velocity. So, replacing values, we get:
L =

L =
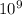 (kg*m^2)/s
(kg*m^2)/s
c) Using the conservation of the linear momentum:
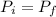
so:
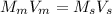
where
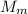 is the mass of the meteor,
is the mass of the meteor,
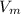 is the velocity of the meteor,
is the velocity of the meteor,
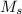 is the mass of the meteor and the space-station after the collition and
is the mass of the meteor and the space-station after the collition and
 is the velocity of the meteor and the space-station after the collition. So, replacing values, we get:
is the velocity of the meteor and the space-station after the collition. So, replacing values, we get:
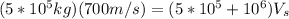
Solving for
 :
:
 = 233.3m/s
= 233.3m/s
d) Using the conservation of the angular momentum:
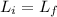
so:
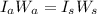
where
 is the moment of inertia of the station,
is the moment of inertia of the station,
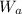 is the angular velocity of the station,
is the angular velocity of the station,
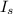 is the moment of inerta of the meteor and the space-station after the collition and
is the moment of inerta of the meteor and the space-station after the collition and
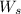 is the angular velocity of the meteor and the space-station after the collition. So, replacing values, we get:
is the angular velocity of the meteor and the space-station after the collition. So, replacing values, we get:
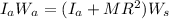

solving for
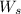 :
:
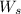 = 0.1 rad/s
= 0.1 rad/s